Reverberation Time: RT10, RT15, RT20, RT30, RT60???
by Richard Honeycutt
W. C. Sabine defined reverberation time (RT) as the time required for a sound having an SPL of 60 dB to decay to the threshold of audibility. What about RT10, RT15, RT20, RT30?
In his original papers, W. C. Sabine defined reverberation time (RT) as the time required for a sound having an SPL of 60 dB to decay to the threshold of audibility. Early tests were made using a pistol firing blanks, an electric spark source, or bursting balloons.
Today, there are a variety of ways to measure RT using computers or dedicated measuring devices. All of these share the need for the sound level to be well above the background noise level. Thus, if the HVAC system in a room produces a 40 dB noise level, the initial sound used to measure RT must be well above 100 dB, so that the lower end of the decay will not be buried in noise. Actually, a 10- or 20-dB margin is better. Therefore, to facilitate practical measurements, standards have been issued that allow extrapolation of RT based upon measurement of only part of the decay. This approach works, because in a diffuse room (the only kind where Sabine’s approach will work), the decay curve in dB/second is linear.
A similar situation prevails in computerized acoustical modeling, except that in this case, the culprit is not the background noise, but the computation time required.
The various RT measures are stated as “RTXX”, where XX is a number of dB. For example, RT20 is the RT value extrapolated from the time required for sound to decay by 20 dB,from -5 dB to- 25 dB. The initial 5 dB of decay is ignored, because in many rooms, it consists mainly of early reflections, and not true reverberation, thus spoiling the uniformity of the slope. So if the time required for sound to decay by 20 dB (from -5 to -25 dB) is 400 ms, we can extrapolate to find RT by multiplying 400 ms by 3, giving 1.2 seconds, since 20 dB is 1/3 of 60 dB, and the decay curve is presumed to be linear.
Figure 1
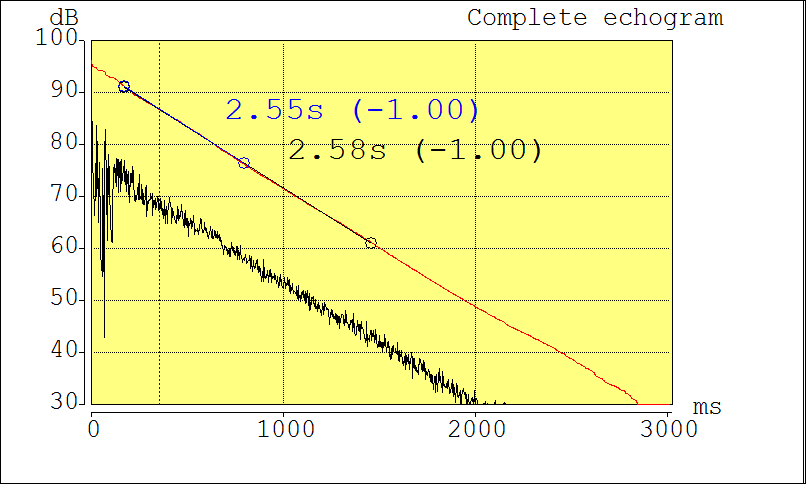
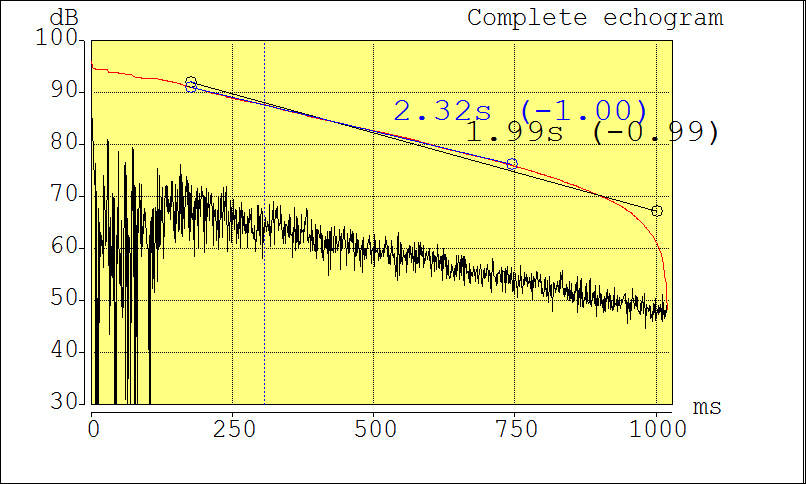
Figure 1 shows a CATT-modeled room using the proper number of rays and a long enough truncation time so that the RT can be accurately calculated. The blue line segment is RT15, and the red one is RT30. The fact that they are almost equal indicates that the room is “Sabine-like”, and that the proper number of rays were used in the analysis. Figure 2 shows the results of analyzing the same room using too few rays and too short a truncation time.
Figure 2
Notice that RT15 and RT30 differ significantly because of incorrect analysis. In this case, the too-short ray truncation time causes the decay to artificially end too early. The use of insufficient rays can cause the end of the reverb tail to be ragged, producing artifacts in the decay curve.
Figure 3
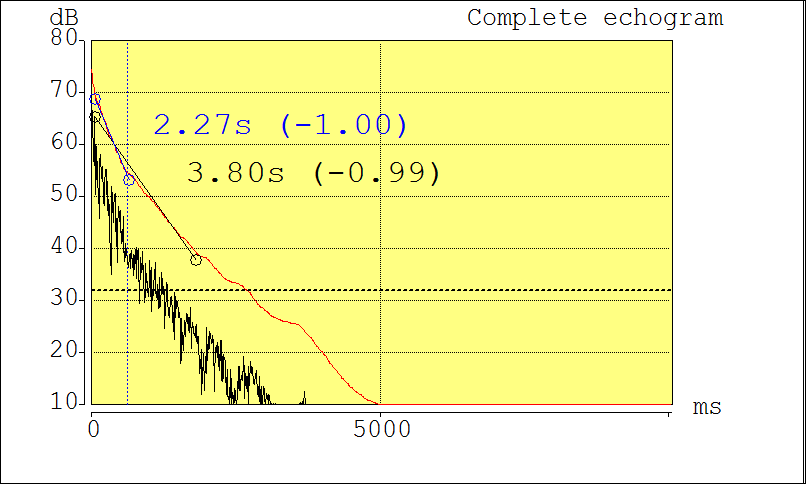
Figure 3 shows the RT of a room consisting of two coupled acoustical spaces. An example of such a room would be an auditorium having a deep under-balcony with a low under-balcony ceiling. Such spaces do not meet the requirements for calculating using the Sabine method. Notice that the T15 and T30 differ considerably, even though the proper number of rays and the correct ray truncation time were used in the analysis. This difference between T15 and T30 results from the concave decay of the room. In fact, in such a room, there may be significant differences in RT from one listener location to another.
A measurement made with too much background noise would give a similar “decay curve”, although it would not truly be a decay curve, but rather a decay-and-noise curve. RT10, RT15, etc. would disagree, and neither would correspond to a true RT for the room. Liberty Instruments Praxis and the NTI Acoustilyzer will not give any results if the background noise is too high, thus preventing errors from this source. Other measurement systems may or may not have such safeguards built in. rh
Richard A. Honeycutt developed an interest in acoustics and electronics while in elementary school. He assisted with film projection, PA system operation, and audio recording throughout middle and high school. He has been an active holder of the First Class Commercial FCC Radiotelephone license since 1969, and graduated with a BS in Physics from Wake Forest University in 1970, after serving as Student Engineer and Student Station Manager at 50-kW WFDD-FM. His career includes writing engineering and maintenance documents for the Bell Telephone System, operating a loudspeaker manufacture company, teaching Electronics Engineering Technology at the college level, designing and installing audio and video systems, and consulting in acoustics and audio/video design. He earned his Ph.D. in Electroacoustics from the Union Institute in 2004. He is known worldwide as a writer on electronics, acoustics, and philosophy. His two most recent books are Acoustics in Performance and The State of Hollow-State Audio, both published by Elektor.